The world has shifted,
Yet still, I am who I am
Oath of symmetry
最も基礎的な量子伝導に異常ホール効果があります。19世紀後半に発見されて以来、異常ホール効果は強磁性体において磁化に比例して現れることが経験的に知られてきました。言い換えれば、磁化を持たない磁性体、たとえば、スピン液体や反強磁性体において、異常ホール効果は現れないと考えられてきました。しかし、近年、その起源に対する理解が進み、これらの磁性体においても、異常ホール効果が出現すること、また、その起源は電子構造のトポロジカルな性質が起源であることがわかってきました。
異常ホール効果は1880年に当時Johns Hopkins大学の博士課程の学生であったEdwin Hallが発見して以来、その理論的理解が完成するまでには1世紀以上もの時間を要しました。20世紀中ごろには、Karplus Luttingerによる内因機構、次いで、スキュー散乱やサイドジャンプという外因機構が提案され、それらの間で長らく論争が起こりました。その混沌とした状況に解決の糸口をもたらしたのが、1982年に量子ホール効果の研究で提案されたTKNN(Thouless - Kohmoto - Nightingale - den Nijs)の式であり、それ以降に続くベリー位相の概念による定式化です。2016年、Thouless氏はこの功績でノーベル物理学賞を受賞しています。このFormalismが強磁性金属の異常ホール効果に拡張されたのが、20世紀の終わりから21世紀の初頭にかけてです。これによると、異常ホール効果は、もはや、磁化によるものではなく、逆格子空間に存在するベリー曲率によるものであると理解できます。このベリー曲率は異常ホール効果を誘起する、まさに実空間の磁場と透過な役割を担うことから、仮想磁場とも呼ばれています。通常、仮想磁場は磁化に比例するために、異常ホール効果はこれまで磁化の大きな強磁性体でのみ実験的に観測されてきました。一方、近年の理論的な発展により、反強磁性やスピン液体においても、大きなベリー曲率による異常ホール効果が出現する可能性が指摘されていましたが、実現には至っていませんでした。
そのなか、我々のグループは、一世紀以上の常識を破り、磁化がなくても異常ホール効果が現れることをスピン液体[1]や反強磁性体[2,3]における実験により世界で初めて明らかにしてきました。特に、反強磁性体においては、強磁性体の異常ホール効果と同程度かそれを凌ぐような大きなホール伝導率が観測されています[2,3]。さらに、我々の研究から、トポロジカルな粒子であるワイル粒子に由来した、運動量空間での数100テスラ級の仮想磁場が、この巨大ホール効果を誘起していることを明らかにしました[4]。また、その後の理論的考察からこの仮想磁場の発現には反強磁性体の有する多極子が本質的に重要であることも分かってきました[5]。これらの発見を契機に、反強磁性体で次々と新しい現象の開拓が可能となり、異常ホール効果に加えて、熱、光とカップルすることにより生ずる異常ネルンスト効果[6]、磁気光学効果[7]、カイラル異常[4,8]、スピン軌道トルクによるスイッチング[8,9]など新しい現象を世界に先駆けて続々と報告してきました。
さらに近年では反強磁性体以外の磁性体においても、トポロジカルな電子状態が見つかってきています。例えばCo2MnGaは室温で強磁性状態ですが、バンド構造を調べると量子リフシッツ転移に近い傾いたワイルコーンを持つことがわかりました[10]。また同じく強磁性体のFe3X (X = Ga, Al)ではノーダルウェブ(対称性で守られたノーダルラインが平坦バンド上に密集した構造)がフェルミエネルギー近傍にあることがわかりました[11]。これらの物質ではトポロジカルな電子構造に由来した巨大ベリー曲率により、巨大異常ネルンスト効果が見つかっています。異常ネルンスト効果は温度勾配と磁化に垂直方向に熱起電力が発生する現象であり、従来の熱電効果(ゼーベック効果)には無かった様々なメリットが考えられています。例えば、薄膜を使った高感度かつ安価な熱流センサーが既に実現しています[12,13,14]。
このように、我々が進めるトポロジカルな電子構造に由来する新しい量子伝導現象の研究は、物性物理だけでなく、高エネルギー物理、スピントロニクスや、エネルギーハーベスティングなど、基礎から応用まで幅広い分野に大きな波及効果をもたらしています。
[1] Y. Machida et al., Nature 463, 210 (2010).
[2] S. Nakatsuji, N. Kiyohara, and T. Higo, Nature 527, 212 (2015).
[3] N. Kiyohara, T. Tomita, and S. Nakatsuji, Phys. Rev. Applied 5, 064009 (2016).
[4] K. Kuroda, T. Tomita et al., Nat. Mater. 16, 1090 (2017).
[5] M.-T. Suzuki et al., Phys. Rev. B 95, 094406 (2017).
[6] M. Ikhlas, T. Tomita et al., Nat. Phys. 13, 1085 (2017).
[7] T. Higo et al., Nat. Photon. 12, 73 (2018).
[8] H. Tsai, T. Higo et al., Nature 580, 608 (2020).
[9] T. Higo K. Kondou et al., Nature 607, 474 (2022).
[10] A. Sakai, et al., Nat. Phys. 14, 1119 (2018).
[11] A. Sakai, S. Minami, T. Koretsune, T. Chen, T. Higo et al., Nature 581, 53 (2020).
[12] T. Higo et al., Adv. Funct. Mater. 2008971 (2021).
[13] H. Tanaka, T. Higo et al., Adv. Mater. 2303416 (2023).
[14] R. Uesugi, T. Higo, S. Nakatsuji, Appl. Phys. Lett. 123, 252401 (2023).
トポロジカル量子物性と量子伝導
研究ハイライト
Robust giant anomalous Nernst effect in polycrystalline nodal web ferromagnets
Y. Wang, A. Sakai, S. Minami, H. Gu, T. Chen, Z. Feng, D. Nishio-Hamane, S. Nakatsuji, Appl. Phys. Lett. 125, 081901 (2024).
The transverse thermoelectric effect based on the anomalous Nernst effect (ANE) has attracted attention, especially for thermoelectric and spintronic applications. Fe3X (X = Ga, Al) is known to exhibit a large ANE at room temperature owing to the topological electronic band structure so-called nodal web. Here, we systematically investigate ANE in the polycrystalline Fe3Ga1−xAlx and Fe3Al1−xSix. Despite significant chemical substitutions, a very robust feature of the large ANE −Syx∼ 5.7 μ V/K is found for Fe3Ga1−xAlx (0≲x≲0.6), exhibiting a striking x independent nodal web contribution. Since aluminum is more low-cost and abundant than gallium, our results indicate Fe3Ga1−xAlx (x∼0.6) should be suitable for large-scale thermoelectric applications.

Large spontaneous magneto-thermoelectric effect in epitaxial thin films of the topological kagome ferromagnet Fe3Sn
S. Kurosawa, T. Higo, S. Saito, R. Uesugi, S. Nakatsuji,
Phys. Rev. Materials 8, 054206 (2024). (Editors' Suggestion, Featured in Physics)
Recent intensive studies in metallic kagome systems have revealed various correlated topological and electronic states, leading to their spintronic applications. The kagome ferromagnetic metal Fe3Sn has been found to host a topological nodal plane near the Fermi energy and exhibits large transverse transport responses. Here we report the fabrication of the epitaxial thin films of Fe3Sn and the observation of its large anomalous Nernst effect (ANE). Structural and magnetic measurements reveal (0001)-orientated epitaxial growth of kagome 𝐷019 Fe3Sn and the magnetic easy plane along the substrate plane. Moreover, transport measurements confirm the large ANE of 2.4 µV/K at room temperature, comparable to that of the bulk polycrystalline samples. The obtained Fe3Sn film should be useful for thermoelectric applications, because the binary compound based on inexpensive elements exhibits large spontaneous ANE voltage under an out-of-plane heat current due to the magnetic and electronic states stabilized by the magnetic anisotropy.

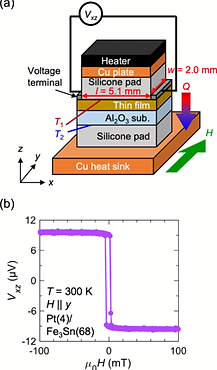
©2024 American Physical Society
Anisotropic anomalous transport in the kagome-based topological antiferromagnetic Mn3Ga epitaxial thin films
M. Raju, R. Romero, III, D. Nishio-Hamane, R. Uesugi, M. Asakura, Z. Tagay, T. Higo, N. P. Armitage, C. Broholm, S. Nakatsuji
Phys. Rev. Materials 8, 014204 (2024). (Editors' Suggestion)
Mn3X (X=Sn, Ge, Ga) kagome Weyl semimetals have attracted significant research interest due to their large anomalous Hall, thermal, and optical effects originating from their nontrivial band topology. These large topological effects together with the antichiral antiferromagnetic order that can be manipulated through various experimental means provide unique platforms for developing high-speed spintronics. Mn3Ga is known to have the largest Néel temperature (TN≈480 K), which is useful for developing antiferromagnetic spintronics. Here, we establish the epitaxial growth of antiferromagnetic Mn3Ga films by magnetron sputtering and present their structure, magnetotransport, terahertz properties, and exchange bias effect in Mn3Ga/NiFe bilayers, establishing their remarkable properties essential for future investigations towards device applications.

©2024 American Physical Society
Giant anomalous Nernst effect in polycrystalline thin films of the Weyl ferromagnet Co2MnGa
R. Uesugi, T. Higo, S. Nakatsuji, Appl. Phys. Lett. 123, 252401 (2023).
Recent discoveries of topological magnets have opened up diverse spintronic applications of large responses due to their unique band structures. A prominent example is the anomalous Nernst effect (ANE), a transverse magneto-thermoelectric phenomenon that produces an electromotive force orthogonal to the heat flux and magnetization. Unlike the Seebeck effect generating an electromotive force parallel to the heat flux, transverse thermoelectric properties of ANE well fit in the lateral configurations of devices fabricated through conventional thin-film fabrication processes. This feature enables distinct device applications through a simplified fabrication process, reduced production cost, extensive area coverage, and enhanced flexibility. In this study, we report the highest ANE ever recorded among all reported polycrystalline films to date by using a topological ferromagnet. In particular, we have fabricated high-quality polycrystalline thin films of the Weyl ferromagnet Co2MnGa that exhibit a large ANE of − 5.4 μV/K. By retaining a high film density, we demonstrate the sizable ANE in the films obtained using a simple fabrication process well suited for device developments. Establishing a thin-film fabrication technique capable of producing a giant ANE facilitates spintronic applications of the Weyl ferromagnet, including diverse ANE-based device applications.

Giant and Robust Anomalous Nernst Effect in a Polycrystalline Topological Ferromagnet at Room Temperature
Z. Feng, S. Minami, S. Akamatsu, A. Sakai, T. Chen, D. Nishio-Hamane, S. Nakatsuji, Adv. Funct. Mater. 32, 2206519 (2022).
Recent discoveries of the topological magnets have opened a new path for developing a much simpler thermoelectric conversion module using the anomalous Nernst effect (ANE). To accelerate such innovation, it is essential to design materials suitable for industrial processes, and thus a high-ANE polycrystalline material has been highly desired. Recently, the giant room-temperature ANE has been reported in single crystals of the topological ferromagnet Fe3Ga. Owning to its cubic structure, the anomalous Hall effect and ANE are isotropic. These properties potentially allow to employ a polycrystalline form of the material to design an ANE-based thermopile. Here, a giant and robust room-temperature ANE in the polycrystalline FexGa4−x (2.96 < x < 3.15) is reported, which can be enhanced up to 5.4 µV K−1; this value hits the highest room-temperature record for polycrystalline magnets. Comparison of the experimental results with the theoretical study of the Fe-doping effect on the transport properties of FexGa4−x reveals that the Fermi energy tuning near the topological nodal-web structure is the key to enhancing the ANE. Moreover, the large value of more than 5.1 µV K−1 is observed for an extended region of composition, confirming the robust characteristics of the topological electronic structure.


© 2022 Wiley-VCH GmbH
Large anomalous Nernst effect and nodal plane in an iron-based kagome ferromagnet
T. Chen, S. Minami, A. Sakai, Y. Wang, Z. Feng, T. Nomoto, M. Hirayama, R. Ishii, T. Koretsune, R. Arita, S. Nakatsuji,
Sci. Adv. 8, eabk1480 (2022).
Anomalous Nernst effect (ANE), converting a heat flow to transverse electric voltage, originates from the Berry phase of electronic wave function near the Fermi energy EF. Thus, the ANE provides a sensitive probe to detect a topological state that produces large Berry curvature. In addition, a magnet that exhibits a large ANE using low-cost and safe elements will be useful to develop a novel energy harvesting technology. Here, we report our observation of a high ANE exceeding 3 microvolts per kelvin above room temperature in the kagome ferromagnet Fe3Sn with the Curie temperature of 760 kelvin. Our theoretical analysis clarifies that a “nodal plane” produces a flat hexagonal frame with strongly enhanced Berry curvature, resulting in the large ANE. Our discovery of the large ANE in Fe3Sn opens the path for the previously unexplored functionality of flat degenerate electronic states and for developing flexible film thermopile and heat current sensors.
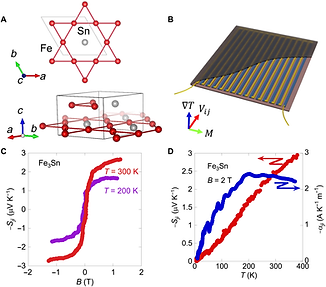
Copyright © 2022 The Authors, some rights reserved (CC BY-NC)
Logarithmic criticality in transverse thermoelectric conductivity of the ferromagnetic topological semimetal CoMnSb
H. Nakamura, S. Minami, T. Tomita, A. A. Nugroho, S. Nakatsuji, Phys. Rev. B 104, L161114 (2021).
We report the results of our experimental studies on the magnetic, transport, and thermoelectric properties of the ferromagnetic metal CoMnSb. A sizable anomalous Hall conductivity σyx and transverse thermoelectric conductivity αyx are found experimentally and comparable in size to the values estimated from first-principles calculation. Our experiment further reveals that CoMnSb exhibits −TlogT critical behavior in αyx(T), deviating from Fermi liquid behavior αyx∼T over a decade of temperature between 10 and 400 K, similar to ferromagnetic Weyl and nodal-line semimetals. Our theoretical calculation for CoMnSb also predicts the −TlogT behavior when the Fermi energy locates near the Weyl nodes in momentum space.
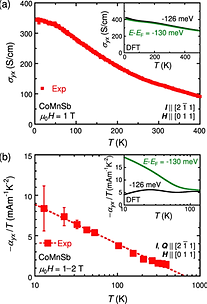
©2021 American Physical Society
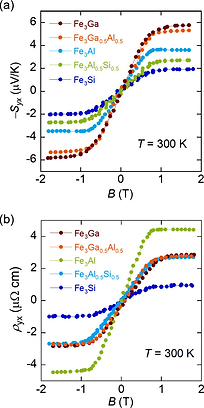
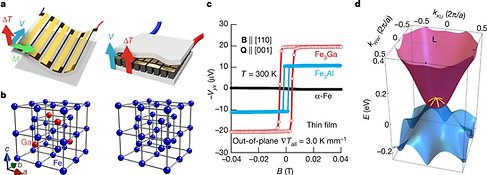
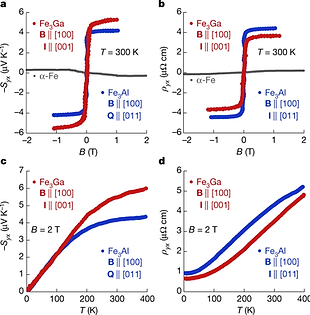
Iron-based binary ferromagnets for transverse thermoelectric conversion
A. Sakai, S. Minami, T. Koretsune, T. Chen, T. Higo, Y. Wang, T. Nomoto, M. Hirayama, S. Miwa, D. Nishio-Hamane, F. Ishii, R. Arita, S. Nakatsuji, Nature 581, 53 (2020).
Thermoelectric generation using the anomalous Nernst effect (ANE) has great potential for application in energy harvesting technology because the transverse geometry of the Nernst effect should enable efficient, large-area and flexible coverage of a heat source. For such applications to be viable, substantial improvements will be necessary not only for their performance but also for the associated material costs, safety and stability. In terms of the electronic structure, the anomalous Nernst effect (ANE) originates from the Berry curvature of the conduction electrons near the Fermi energy. To design a large Berry curvature, several approaches have been considered using nodal points and lines in momentum space. Here we perform a high-throughput computational search and find that 25 percent doping of aluminium and gallium in alpha iron, a naturally abundant and low-cost element, dramatically enhances the ANE by a factor of more than ten, reaching about 4 and 6 microvolts per kelvin at room temperature, respectively, close to the highest value reported so far. The comparison between experiment and theory indicates that the Fermi energy tuning to the nodal web—a flat band structure made of interconnected nodal lines—is the key for the strong enhancement in the transverse thermoelectric coefficient, reaching a value of about 5 amperes per kelvin per metre with a logarithmic temperature dependence. We have also succeeded in fabricating thin films that exhibit a large ANE at zero field, which could be suitable for designing low-cost, flexible microelectronic thermoelectric generators.
© The Author(s), under exclusive licence to Springer Nature Limited 2020
